For the Infinite Slot Determine the Charge Density

B The left end at x0 is closed o with a metal strip at constant potential V 0 as illustrated in the following gure. The beam supports the distributed load shown.

Ch3 特殊技巧 Special Techniques Ppt Download

Who are the experts.

. The infinite slab can be thought of a set of parallel infinite sheets of uniform surface charge density σ ρdy where dy is the thickness of charge sheet. Students also viewed these Electrodynamics questions Infinite sheet A carries a positive uniform charge density and sheet. A is the surface area of the table.

33 determine the charge density o-y on the strip at x 0 assuming it is a conductor at constant potential V 0. For the infinite slot Ex. 33 determine the charge density o-y on the strip at x 0 assuming it is a conductor at constant potential V 0 Example 33 Two infinite grounded metal plates lie parallel to the xz plane one at y 0 the other at y.

33 determine the charge density y on the strip at x 0 assuming it is a conductor at constant potential V0. Determine the charge density y on this strip. Buy a solution for 05.

For the infinite slot Ex. σ ϵ 0 V n E q. A 25 Perform the separation of variables in the Laplaces equation in spherical coordinates assuming that the electric potential depends on the 0.

33 determine the charge density σ y on the strip at x 0 assuming it is a conductor at constant potential V0. Two infinite grounded metal plates lie parallel to the xz plane one at y 0 the other at y a Fig. For the infinite slot Ex.

33 determine the charge density sigmay on the strip al x0 assuming it is a conductor at constant potential V_0. Determine the molecular formulas corresponding to each of the following structures. One strip extends from 0 y a2 and is at a constants potential V 0.

An infinite plate with has a charge density σ. Reference example 33 Two infinite grounded metal plates lie parallel to the xz plane one at y 0 the other at y a Fig. Experts are tested by Chegg as specialists in their subject area.

A 25 For the infinite slot Example 33 on page 127 determine the charge density σ y on the strip at x 0 assuming it is a conductor at constant potential V 0 Problem 3. V V 14 The Image Charge. For the infinite slot Ex.

0 far from the charge. We review their content and use your feedback to keep the quality high. V x y 4 V 0 π n 1 3 5.

Find the potential in the infinite slot of example 33 Griffiths page 127 if the boundary at x 0 is composed of two metal strips. For the infinite slot Ex. 4 9 V x yfrac 4 V_ 0 pi sum_ n135 ldots frac 1 n e -n pi x a sin n pi y a quad text Eq.

33 determine the charge density σ y on the strip at x 0 assuming it is a conductor at constant potential V0. 0 when z 0 since the conducting plane is grounded. For the infinite slot Ex.

SI unit of Linear charge density is coulomb Volume Charge Density. σ q A. For the infinite slot Ex.

321 The Infinite Grounded Conducting Plane The boundary conditions of this case are. 33 determine the charge density y on the strip at x 0 assuming it is a conductor at constant potential V0. Find the potential in this in nite slot.

The bottom of the conductor is grounded. 2 The solution will be in 8 hours. It is also known that A9d2 and the system is stable.

ρ q v. 33 determine the charge density σy on the strip at x 0 assuming it is a conductor at constant potential V0. What is the potential in the region above the plane.

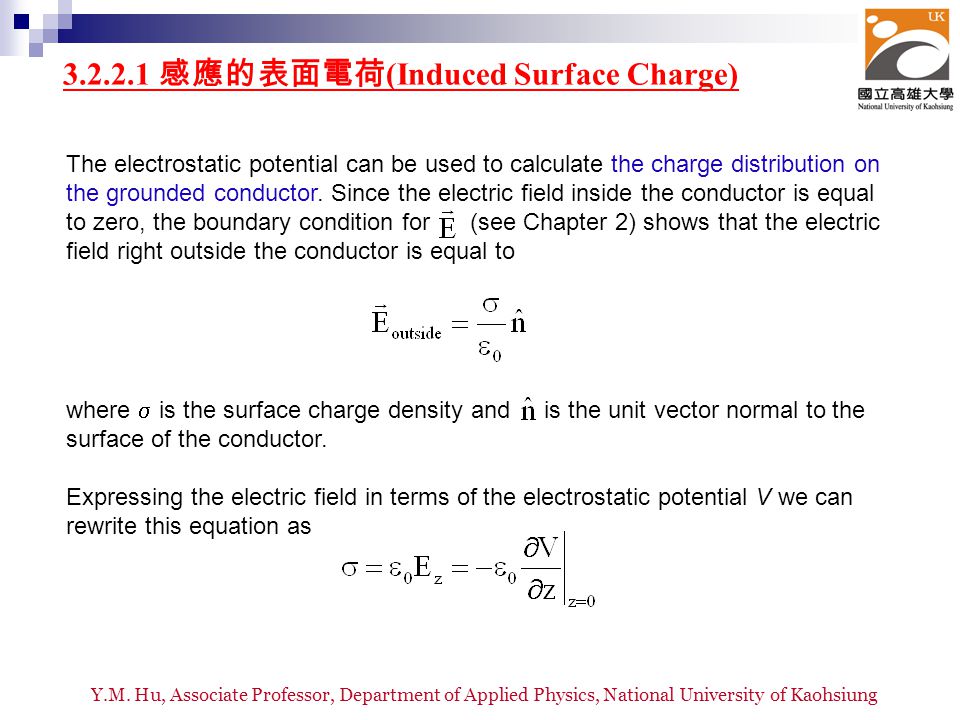
The electrostatic potential in the slot is equal to The charge density at the plate at x 0 can be obtained using the boundary condition for the electric field at a boundary. For the infinite slot determine the charge density on the strip at x0 assuming it is a conductor at constant Vo. Where q is the charge and A is the area over which it is distributed.

33 determine the charge density o-y on the strip at x 0 assuming it is a conductor at constant potential V 0 Example 33 Two infinite grounded metal plates lie parallel to the xz plane one at y 0 the other at y. For the infinite slot Ex. 1 You can buy this solution for 05.

The field for such a sheet independent x and z and normal to the charge sheet therefore normal to the x-z plane for σ0 E points away from the plane of charge. For the infinite slot Ex. We move a conductor under it as described in the figure below.

SI unit of Linear charge density is coulomb Solved Example. Find the charge density at the top of the conductor the part marked with an A on it Relevant Equations. 33 determine the charge density σy on the strip at x 0 assuming it is a conductor at constant potential V 0.

Video answers for all textbook questions of chapter 3 Special Techniques Introduction to Electrodynamics by Numerade. The other strip extends from a2 y a and is at a constant potential of -V 0. For the infinite slot Example 33 Griffiths determine the charge density on the strip at x0 assuming it is a conductor at constant potential.

1 n e n π x a sin n π y a E q. Determine the resultant internal loadings. Gri t problem 312 313 2.

Suppose a point charge is held a distance d above an infinite grounded conducting plane. 3 If you want the solution will be free for all following visitors. For the infinite slot Ex.

A Show that the average field due to a single charge q at point r inside the sphere is the same as the field at r due to a uniformly charged sphere with p qr R3 namely rR3J 42 where is the vector from r to b The latter can be. Where q is the charge and V is the volume over which it is distributed. Find the charge density if a charge of 8 C is present in a cube of 4.

Chapter 3 Special Techniques For Calculating Potentials

Chapter 3 Special Techniques For Calculating Potentials

2